Lens aperture
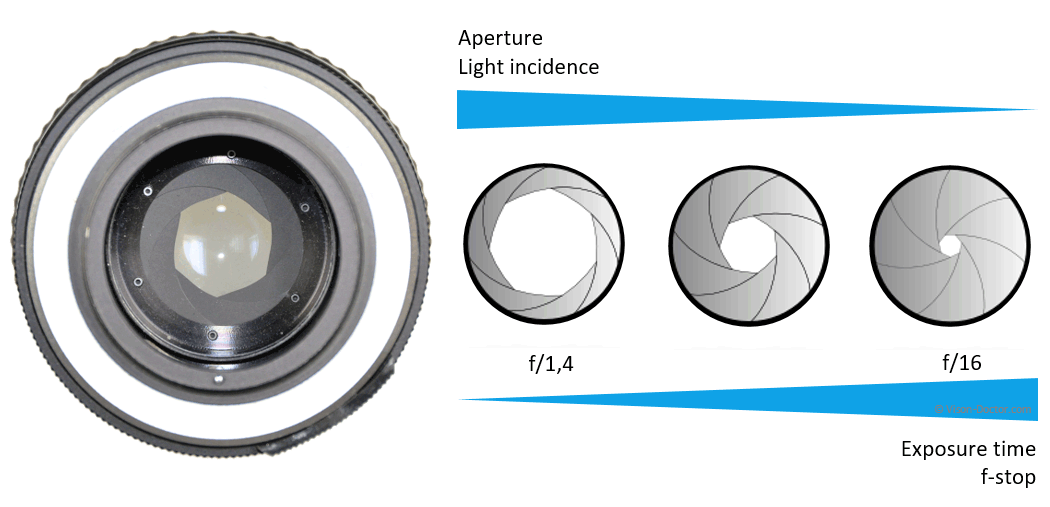
The aperture of the optics corresponds to the aperture of the lens through which light can fall on the sensor. Therefore it is directly responsible for the image brightness. The size of the aperture is achieved by a circular arrangement of overlapping blades, the function of which corresponds to the pupil of the human eye. This is done by radially limiting the optical path from the edge of the lens.
Changing the aperture affects the
A higher focal ratio results in a longer exposure time, however, it generates a high depth of field in the image; a widely open aperture causes short sensor exposure times, but also a low depth of field.
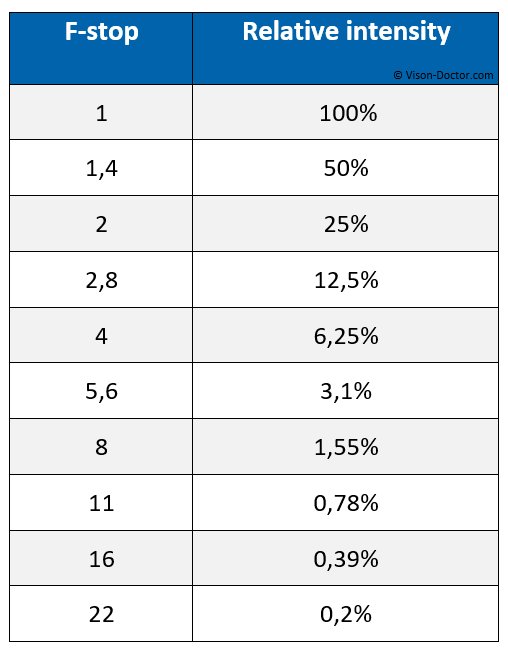 |
The international aperture scale is arranged in such a way that each step means halving or doubling the exposure time.
 |
The diaphragm blades reduce the opening diameter of the optics by the factor 1.4 (square root of 2), corresponding to halving the opening surface.
| 1.4 * 1.4 = 2 | 2 * 1.4 = 2.8 | 2.8 * 1.4 = 4 etc. |
The result is the international f-stop series:
f/1--f/1 0.4 - f / 2 - f/2.8 - f / 4--f/5.6--f/8--f/1--f/16-f / 22nd ..
The absolute diameter of the diaphragm depends on the focal length of the optics. In case of a lens with f´=50mm, a diaphragm open by 12.5 mm has the same effect as a diaphragm open by 25 mm on optics with a focal length of 100 mm. If the diameter of the aperture is divided by the focal length, the result in both cases of our example is 1/4, independent of the focal length.
Therefore the indication of the aperture is often given as a fraction of the focal length and not as an absolute value in millimetres, they are referred to as f-numbers or f-stops. Lens indications of 1/4 are also written as f/4, F4 or 1:4.
Effective focal ratio
The values of the focal ratio indicated on the lens are normally calculated for an image from the infinite. However, in case of images from a short distance, which is rather common in machine vision, it becomes apparent that the image is darker. In case of macro-images, this effect is particularly obvious.
keffective = k * (1 + β)
The image scale β is calculated from
β = image / object
In case of an image of 144 mm ("postcard size" as object field) on a 1/3" camera chip with 4.8 mm, the result is β = 1/30, i.e. β does not carry much weight in the above formula. Using very small object fields and comparatively large sensors, the effective focal ratio can vary strongly. The picture appears clearly darker.
Tip:An online wizard for the calculation of the effective focal ratio can be found in the "Service" area of this website.
Important for imaging
By cutting off marginal rays (artificial vignetting of the optics), certain imaging errors like chromatic longitudinal errors, spherical aberration, coma and astigmatism are reduced when stopping down. In this way the quality of the optic image is enhanced, as focus differences and other blurred imaging forms are avoided.
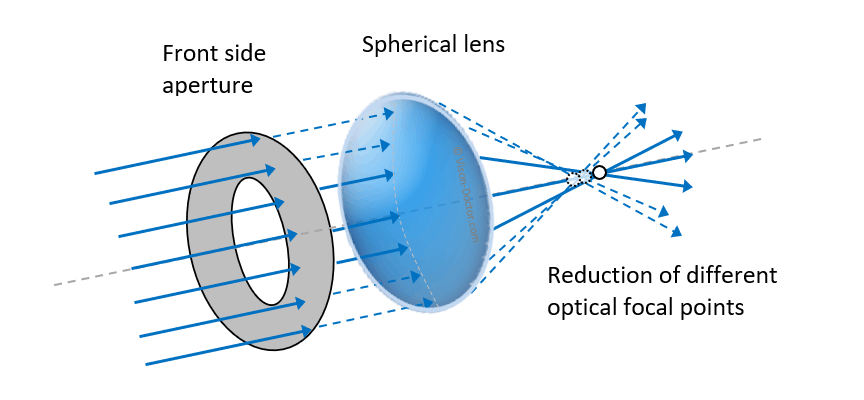 |
Optimum imaging results are mostly achieved using an aperture of 5.6 to 8 in case of entocentric lenses. Further closing of the aperture intensifies the increasing effect of light diffraction at the mechanic slit of the diaphragm, which in turn may result in a reduced sharpness of the image.







